|
Prolegomena to an Apology for
Pragmaticism - 1906 |
| |
|
530. |
Come on, my Reader, and let us construct a diagram to
illustrate the general course of thought; I mean a
system of diagrammatization by means of which any course
of thought can be represented with exactitude.
"But why do that, when the thought itself is present to
us?" Such, substantially, has been the interrogative
objection raised by more than one or two superior
intelligences among whom I single out an eminent and
glorious General.
Recluse that I am, I was not ready with the
counter-question, which should have run, "General, you
make use of maps during a campaign, I believe. But why
should you do so, when the
country they represent is right there?" Thereupon,
had he replied that he found details in the maps
that were so far from being "right there," that they
were within the enemy's lines, I ought to have pressed
the question, "Am I right, then
,in understanding that, if you were thoroughly
and perfectly familiar with the country, as, for
example, if it lay just about the scenes of your
childhood, no map of it would then be of the smallest
use to you in laying out your detailed plans?" To that
he could only have rejoined, "No, I do not say that,
since I might probably desire the maps to stick pins
into, so as to mark each anticipated day's change in the
situations of the two armies." To that again, my sur-rejoinder
should have been, "Well, General, that precisely
corresponds to the advantages of a diagram of the course
of a discussion. Indeed, just there, where you have so
clearly pointed it out, lies the advantage
of diagrams in general. Namely, if I may try to state
the matter after you, one can make exact experiments
upon uniform diagrams; and when one does so, one must
keep a bright lookout for unintended and unexpected
changes thereby brought about in the relations of
different significant parts of the diagram to one
another. Such operations upon diagrams, whether external
or imaginary, take The place of the experiments upon
real Things That one performs in Chemical and physical
research. Chemists have ere now, I need not say,
described experimentation as The putting of questions to
Nature. Just so, experiments upon diagrams are questions
put to The Nature of The relations concerned. " The
General would here, may be, have suggested (if I may
emulate illustrious warriors in reviewing my encounters
in afterthought), that there is a good deal of
difference between experiments like the Chemist's, Which
are trials made upon The very substance whose behavior
is in question, and experiments made upon diagrams,
these latter having no physical connection with the
things they represent. The proper response to that, and
the only proper one, making a point that a novice in
logic would be apt to miss, would be this: "You are
entirely right in saying that the chemist experiments
upon the very object of investigation albeit, after the
experiment is made, the particular sample he operated
upon could very well be thrown away, as Having no
further interest. For it was not the particular sample
that the chemist was investigating; it was the molecular
structure. Now he was long ago in possession of
overwhelming proof that all samples of the same
molecular structure react chemically in exactly the same
way; so that one sample is all one with another But the
object of the chemist's research, that upon which he
experiments, and to which the question he puts to Nature
relates is the Molecular structure, which in aIl his
samples has as complete an identity as it is in the
nature of Molecular Structure ever to possess.
Accordingly, He does, as you say experiment upon the
Very Object under investigation But if you stop a moment
to consider it, you will acknowledge I think that you
slipped in implying that it is otherwise with
experiments made upon diagrams. For what is there the
Object of Investigation? It is the form of a relation
Now this Form of Relation is the very form of the
relation between the two corresponding parts of the
diagram. For example, let fi and f2 be the two distances
of the two foci of a lens from the lens. Then,
1/f1 + 1/f2 = 1/f0
This equation is a diagram of the form of the relation
between the two focal distances and the principal focal
distance; and the conventions of algebra (and all
diagrams, nay all pictures, depend upon conventions) in
conjunction with the writing of the equation, establish
a relation between the very letters f1, f2, f0
regardless of their significance, the form of which
relation is the Very Same as the form of the relation
between the three focal distances that these letters
denote. This is a truth quite beyond dispute. Thus, this
algebraic Diagram presents to our observation the very,
identical object of mathematical research, that is, the
Form of the harmonic mean which the equation aids one to
study. (But do not let me be understood as saying that a
Form possesses, itself, Identity in the strict sense;
that is, what the logicians, translating
ariqmo,
call 'numerical identity.') |
| |
|
|
| 531. |
Not only is it
true that by experimentation upon some diagram an
experimental proof can be obtained of every necessary
conclusion from any given Copulate of Premisses but,
what is more, no "necessary" conclusion is any more
apodictic than inductive reasoning becomes from the
moment when experimentation can be multiplied ad libitum
at no more cost than a summons before the imagination. I
might furnish a regular proof of this, and am dissuaded
from doing so now and here only by the exigency of
space, the ineluctable length the requisite
explanations, and particularly by the present
disposition of logicians to accept as sufficient F. A.
Lange's persuasive and brilliant, albeit defective and
in parts even erroneous, apology for it.
(*) Under these circumstances, I will content
myself with a rapid sketch of my proof. First, an
analysis of the essence of a sign, (stretching that word
to its widest limits, as anything which, being
determined by an object, determines an interpretation to
determination, through it, by the same object), leads to
a proof that every sign is determined by
its object, either first, by partaking in the characters
of the object, when I call the sign an Icon; secondly,
by being really and in its individual existence
connected with the individual object, when I call the
sign an Index:; thirdly, by more or less approximate
certainty that it will be interpreted as denoting the
object, in consequence of a habit (which term I use as
including a natural disposition), when I call the sign a
Symbol. I next examine into the different efficiencies
and inefficiencies of these three kinds of signs in
aiding the ascertainment of truth. A Symbol incorporates
a habit and is indispensable to the application of any
intellectual habit at least. Moreover Symbols afford the
means of thinking about thoughts in ways in which we
could not otherwise think of them. They enable us, for
example, to create Abstractions, without which we should
lack a great engine of discovery. These enable us to
count; they teach us that collections are individuals
(individual = individual object), and in many respects
they are the very warp of reason. But since symbols rest
e. exclusively on habits already definitely formed but
not furnishing any observation even of themselves, and
since knowledge is habit, they do not enable us to add
to our knowledge even so much as a necessary consequent,
unless by means of a definite preformed habit. Indices,
on the other hand, furnish positive assurance of the
reality and the nearness of their Objects. But with the
assurance there goes no insight into the nature of those
Objects. The same Perceptible may, however, function
doubly as a sign. That footprint that Robinson Crusoe
found in the sand, and which has been stamped in the
granite of fame, was an Index to him that some creature
was on his island, and at the same time, as a Symbol,
called up the idea of a man. Each Icon partakes of some
more or less overt character of its Object. They, one
and all, partake of the most overt character of all lies
and deceptions - their Overtness. Yet they have more to
do with the living character of truth than have either
Symbols or indices. The Icon does not stand
unequivocally for this or that existing thing, as the
Index does. Its Object may be a pure fiction, as to its
existence. Much less is its Object
(n1)
necessarily a thing of a sort habitually met with. But
there is one assurance that the Icon does afford in the
highest degree. Namely, that which is displayed before
the mind's gaze--the Form of the Icon, which is also its
object--must be logically possible. This division of
Signs is only one of ten different divisions of Signs
which I have found it necessary more especially to
study. * I do not say that they are all satisfactorily
definite in my mind. They seem to be all trichotomies,
which for an attribute to the essentially triadic nature
of a sign. I mean because three things are concerned in
the functioning of a Sign; the Sign itself, its Object,
and its Interpretant. I cannot discuss all these
divisions in this article; and it can well be believed
that the whole nature of reasoning cannot be fully
exposed from the consideration of one point of view
among ten. That which we can learn from this division is
of what sort a Sign must be to represent the sort of
object that reasoning is concerned with. Now reasoning
has to make its conclusion manifest. Therefore, it must
be chiefly concerned with forms, which are the chief
objects of rational insight. Accordingly, Icons are
specially requisite for reasoning. A Diagram is mainly
an Icon, and an Icon of intelligible relations. It is
true that what must be is not to be learned by simple
inspection of anything. But precisely what we do mean is
that the conclusion follows from the form of the
relations set forth in the premiss. Now since a diagram,
though it will ordinarily have Symboloide Features, as
well as features approaching the nature of Indices, is
nevertheless in the main an Icon of the forms of
relations in the constitution of its Object, the
appropriateness of it for the representation of
necessary inference is easily seen. |
| |
|
|
| |
2. COLLECTIONS |
| 532. |
But since you
may, perhaps, be puzzled to understand how an Icon can
exhibit a necessity--a Must-be--I will here give, as an
example of its doing so, my proof that the single
members of no collection or plural, are as many as
are the collections it includes, each reckoned as a
single object, or, in other words, that there can be no
relation in which every collection composed of members
of a given collection should (taken collectively as a
single object) stand to some member of the latter
collection to which no other such included collection so
stands. This is another expression of the following
proposition, namely: that, taking any collection or
plural, whatsoever, be it finite or infinite, and
calling this the given collection; and considering all
the collections, or plurals, each of which is composed
of some of the individual members of the given
collection (but including along with these Nothing which
is to be here regarded as a collection having no members
at all; and also including the single members of the
given collection, conceived as so many collections each
of a single member), and calling these the involved
collections; the proposition is that there is no
possible relation in which each involved collection
(considered as a single object), stands to a member of
the given collection, without any other of the involved
collections standing in the same relation to that same
member of the given collection. This purely symbolic
statement can be rendered much more perspicuous by the
introduction of Indices, as follows. The proposition is
that no matter what collection C may be, and no matter
what relation R may be, there must be some collection,
c', composed exclusively of members of C, which does not
stand in the relation R to any member, k, of C, unless
some other collection, c", likewise composed of members
of C, stands in the same relation R to the same k. The
theorem is important in the doctrine of multitude, since
it is the same as to say that any collection, no matter
how great, is less multitudinous than the collection of
possible collections composed exclusively of members of
it; although formerly this was assumed to be false of
some infinite collections. The demonstration begins by
insisting that, if the proposition be false, there must
be some definite relation of which it is false. Assume,
then, that the letter R is an index of any one such
relation you please. Next divide the members of C into
four classes as follows: |
| |
Class I is to consist of all
those members of C (if there be any such) to each of
which no collection of members of C stands in the
relation R.
|
| |
Class II is to consist of all those members of C
to each of which one and only one collection of
members of C stands in the relation R; and this
class has two sub-classes, as follows:
|
| |
Sub-Class 1 is to consist of whatever members
of Class II there may be, each of which is
contained in that one collection of members of C
that is in the relation R to it.
Sub-Class 2 is to consist of whatever members of
Class II there may be, none of which is
contained in that one collection of members of C
that is in the relation R to it.
|
| |
Class III is to consist of all those members of
C, if there be any such, to each of which more than
one collection of members of C are in the relation
R.
|
| |
This division
is complete; but everybody would consider the easy
diagrammatical proof that it is so as needless to the
point of nonsense, implicitly relying on a Symbol in his
memory which assures him that every Division of such
construction is complete. I ought already to have
mentioned that, throughout the
enunciation and demonstration of the proposition to be
proved the term "collection included in the given
collection" is to be taken in a peculiar sense to be
presently defined. It follows that there is one
"possible collection" that is included in every other,
that is, which excludes whatever any other excludes.
Namely, this is the ''possible collection'' which
includes only the Sphinxes, which is the same that
includes only the Basilisks, and is identical with the
"possible collection" of all the Centaurs, the unique
and ubiquitous collection called "Nothing," which has no
member at all. If you object to this use of the term
"collection," you will please substitute for it,
throughout the enunciation and the demonstration, any
other designation of the same object. I prefix the
adjective "possible," though I must confess it does not
express my meaning, merely to indicate that I extend the
term "collection" to Nothing, which of course, has no
existence. Were the suggested objection to be persisted
in by those soi-disant reasoners who refuse to think at
all about the object of this or that description, on
the ground that it is "inconceivable," I should not stop
to ask them how they could say that, when that involves
thinking of it in the very same breath, but should
simply say that for them it would be necessary to except
collections consisting of single individuals. Some of
these mighty intellects refuse to allow the use of any
name to denote single individuals, and also plural
collections along with them; and for them the
proposition ceases to be true of pairs. If they would
not allow pairs to be denoted by any term that included
all higher collections the proposition would cease to be
true of triplets and so on In short, by restricting the
meaning of "possible collection' the proposition may be
rendered false of small collections. No general formal
restriction can render it false of greater collections.
I shall now assume that you will permit me to use the
term "possible collection" according to the following
definition. A "possible collection" is an ens
rationis of such a nature that the definite plural
of any noun, or possible noun of definite signification,
(as "the A's," "the B's," etc. ) denotes one and only
one, "possible collection" in any one perfectly definite
state of the universe; and there is a certain relation
between some "possible collections," expressed by saying
that one "possible collection" includes another (or the
same) "possible collection," and if, and only if, of two
nouns one is universally and affirmatively predicable of
the other in any one perfectly definite state of the
universe, then the "possible collection" denoted by the
definite plural of the former includes whatever
"possible collection" is included by the "possible
collection" denoted by the definite plural of the
latter, and of any two different "possible collections,"
one or other must include something not included by the
other.A diagram of the
definition of "possible collection" being compared with
a diagram embracing whatever members of subclasses I and
2 that it may, excluding all the rest, will now assure
us that any such aggregate is a possible collection of
members of the class C, no matter what individuals of
Classes I and III be included or excluded in the
aggregate along with those members of Class II, if any
there be in the aggregate.
We shall select, then, a single
possible collection of members of C to which we give the
proper name, c, and this possible collection shall be
one which contains no individual of Subclass t, but
contains whatever individual there may be of subclass 2.
We then ask whether or not it is true that c stands in
the relation R to a member of C to which no other
possible collection of members of C stands in the same
relation; or, to put this question into a more
convenient shape, we ask, Is there any member of the
Class C to which c and no other possible collection of
members of C stands in the relation R? If there be such
a member or members of C, let us give one of them the
proper name T Then T must belong to one of our four
divisions of this class. That is,
either T belongs to Class I
(but that cannot be, since by the definition of
Class I, to no member of this class is any possible
ollection o members of C in the relation R); or T
belongs to Subclass I (but that cannot be,since by
the definition of that subclass, every member of it
is a member of the only possible collection of
members of C that is R to it, which possible
collection cannot be c, because c is only known to
us by a description which forbids its containing any
member of Subclass I. Now it is c, and c only that
is in the relation R to T);
or T belongs to Subclass 2
(but that cannot be, since by the definition of that
subclass, no member of it is a member of the only
possible collection of members of C that is R to it
which possible collection cannot be c, because the
description by which alone c can be recognized makes
it contain every member of Subclass 2. Now it is c
only that is in the relatiOn R to T);
or T belongs to Class III (but
this cannot be, since to every member of that class,
by the definition of it, more than one collection of
members of C stand in the relation R, while to T
only one collection, namely, c, stands in that
relation).
Thus T belongs to none of the
classes of members of C, and consequently is not a
member of C. Consequently, there is no such member of C;
that is, no member of C to which c, and no other
possible collection of members of C stands in the
relation R. But c is the proper name we were at liberty
to give to whatever possible collection of members of C
we pleased. Hence, there is no possible collection of
members of C that stands in the relation R to a member
of the class C to which no other possible collection of
members of C stands in this relation R. But R is the
name of any relation we please, and C is any class we
please. It is, therefore, proved that no matter what
class be chosen, or what relation be chosen, there will
be some possible collection of members of that class (in
the sense in which Nothing is such a collection) which
does not stand in that relation to any member of that
class to which no other such possible collection stands
in the same relation. |
|
| |
3. GRAPHS AND SIGNS |
| 533. |
When I was a
boy, my logical bent caused me to take pleasure in
tracing out upon a map of an imaginary labyrinth one
path after another in hopes of finding my way to a
central compartment. The operation we nave just gone
through is essentially of the same sort, and if we are
to recognize the one as essentially performed by
experimentation upon a diagram, so must we recognize
that the other is performed. The demonstration just
traced out brings home to us very strongly, also, the
convenience of so constructing our diagram as to afford
a clear view of the mode of connection of its parts, and
of its composition at each stage of our operations upon
it. Such convenience is obtained in the diagrams of
algebra. In logic, however,
the desirability of convenience in threading our way
through complications is much less than in mathematics,
while there is another desideratum which the
mathematician as such does not feel. The mathematician
wants to reach the conclusion, and his interest in the
process is merely as a means to reach similar
conclusions. The logician does not care what the result
may be; his desire is to understand the nature of the
process by which it is reached. The mathematician seeks
the speediest and most abridged of secure methods; the
logician wishes to make each smallest step of the
process stand out distinctly, so that its nature may be
understood. He wants his diagram to be, above all, as
analytical as possible. |
| |
|
|
| 534. |
In view of
this, I beg leave, Reader, as an Introduction to my
defence of pragmatism, to bring before you a very simple
system of diagrammatization of propositions which I
term the System of Existential Graphs. For, by means of
this, I shall be able almost immediately to deduce some
important truths of logic, little understood hitherto,
and closely connected with the truth of pragmaticism;(n1)
while discussions of other points of logical doctrine,
which concern pragmaticism but are not directly settled
by this system, are nevertheless much facilitated by
reference to it. |
| |
|
|
| 535. |
By a graph (a
word overworked of late years), I, for my part,
following my friends Clifford(*)
and Sylvester,(**)
the introducers of the term, understand in general a
diagram composed principally of spots and of lines
connecting certain of the spots. But I trust it will be
pardoned to me that, when I am discussing Existential
Graphs, without having the least business with other
Graphs, I often omit the differentiating adjective and
refer to an Existential Graph as a Graph simply. But you
will ask, and I am plainly bound to say, precisely what
kind of a Sign an Existential Graph, or as I abbreviate
that phrase here, a Graph is. In order to answer this I
must make reference to two different ways of dividing
all Signs. It is no slight task, when one sets out from
none too clear a notion of what a Sign is - and you
will, I am sure, Reader, nave noticed
that my definition of a Sign is not convincingly
distinct--to establish a single vividly distinct
division of all Signs. The one division which I have
already given has cost more labor than I should care to
confess. But I certainly could not tell you what sort of
a Sign an Existential Graph is, without reference to two
other divisions of Signs. It is true that one of these
involves none but the most superficial considerations,
while the other, though a hundredfold more difficult,
resting as it must for a clear comprehension of it upon
the profoundest secrets of the structure of Signs, yet
happens to be extremely familiar to every student of
logic. But I must remember, Reader, that your
conceptions may penetrate far deeper than mine; and it
is to be devoutly hoped they may. Consequently, I ought
to give such hints as I conveniently can, of my notions
of the structure of Signs, even if they are not strictly
needed to express my notions of Existential Graphs. |
| |
|
|
536. |
I have already
noted that a Sign has an Object and an Interpretant, the
latter being that which the Sign produces in the
Quasi-mind that is the Interpreter by determining the
latter to a feeling, to an exertion, or to a Sign, which
determination is the Interpretant. But it remains to
point out that there are usually two Objects, and more
than two Interpretants. Namely, we have to distinguish
the Immediate Object, which is the Object as the Sign
itself represents it, and whose being is thus dependent
upon the Representation of it in the Sign, from the
Dynamical Object, which is the Reality which by some
means contrives to determine the Sign to its
Representation. In regard to the Interpretant we have
equally to distinguish, in the first place, the
Immediate Interpretant, which is the interpretant as it
is revealed in the right under- standing of the Sign
itself, and is ordinarily called the meaning of the
sign; while in the second place, we nave to take note of
the Dynamical Interpretant which is the actual effect
which the Sign as a Sign really determines. Finally
there is what I provisionally term the Final
Interpretant, which refers to the manner in which the
Sign tends to represent itself to be related to its
Object. I confess that my own conception of this third
interpretant is not yet quite free from mist.(*)
Of the ten divisions of signs which nave seemed
to me to call for my special study,
six turn on the characters of an Interpretant and three
on the characters of the Object.(*)
Thus the division into Icons, Indices, and Symbols
depends upon the different possible relations of a Sign
to its Dynamical Object.(**)
Only one division is concerned with the nature of the
Sign itself, and this I now proceed to state. |
| |
|
|
|
537. |
A common mode
of estimating the amount of matter in a MS. or printed
book is to count the number of words.
(n1) There
will ordinarily be about twenty the's on a page and of
course they count as twenty wards. In another sense of
the word "word," however, there is but one word " the "
in the English language; and it is impassible that this
ward should lie visibly on a page or
be heard in any voice for the reason that it is not a
Single thing or Single event It does not exist; it only
determines things that da exist. Such a definitely
significant Form, I propose to
term a Type.(**)
A Single event which happens once and whose identity is
limited to that one happening
or a Single
object or thing which
is in same single place at any one instant of time, such
event or thing being significant only as occurring just
when and where it does, such as this
or that ward on a
single line of a single page of a single copy of a book,
I will venture to call a Token.
(**)
An indefinite significant character such as a tone of
voice can neither be called a Type nor a Taken. I
propose ta call such a Sign a Tone(**)
In order that a Type may be used, it has to be embodied
in a Taken which shall be a sign of the Type and thereby
of the abject the Type signifies. I propose to
call such a Taken of a Type an Instance of the 'Type.
Thus there may be twenty Instances of
the Type "the" on a page. The term (Existential) Graph
will be taken in the sense of a Type; and the act of
embodying it in a Graph-Instance will be termed scribing
the Graph (not the Instance), whether the Instance be
written, drawn, or incised. A mere blank place is a
Graph-Instance, and the Blank per se is a Graph - but I
shall ask you to assume that it has the peculiarity that
it cannot be abolished from any Area on which it is
scribed as long as that Area exists. |
| |
|
|
|
538. |
A familiar
logical triplet is Term, Proposition, Argument.(*)
In order to make this a division of all signs, the first
two members have to be much widened. By a Seme(**)
I shall mean anything which serves for any purpose
as substitute for an object of which it is, in some
sense, a representative or Sign. The logical Term, which
is a classname is a Seme. Thus, the term "The mortality
of man" is a Seme. By a Pheme(***)
I mean a Sign which is equivalent to a grammatical
sentence, whether it be Interrogative, Imperative or
Assertory. In any case, such a Sign is intended to
have some sort of compulsive
effect on the Interpreter of it As the third member of
the triplet, I sometimes use the word Delome (pronounce
deeloam, from deloma(Gr)), though Argument would answer
well enough. It is a Sign which has the Form of tending
to act upon the Interpreter through his own self control
representing a process of change in thoughts or signs as
if to induce this change in
the Interpreter. A Graph is a Pheme, and in my use
hitherto, at least a proposition. An Argument is
represented by a series of Graphs. |
| |
|
|
| |
4. UNIVERSES AND PREDICAMENTS |
|
539. |
The Immediate
Object of all knowledge and all thought is, in the last
analysis, the Percept. This doctrine in no wise
conflicts with Pragmaticism which holds that the
Immediate Interpretant of all thought proper is Conduct.
Nothing is more indispensable to a sound epistemology
than a crystal-clear discrimination
between the Object and the Interpretant of knowledge;
very much as nothing is more indispensable to sound
notions of geography than a crystal-clear discrimination
between north latitude and south latitude; and the one
discrimination is not more rudimentary than the other.
That we are conscious of our Percepts is a theory that
seems to me to be beyond dispute; but it is not a fact
of Immediate Perception. A fact of Immediate Perception
is not a; Percept nor any part of a Percept; a Percept
is a Seme, while a fact of Immediate Perception or
rather the Perceptual Judgment of which such fact is the
Immediate Interpretant, is a Pheme that is the direct
Dynamical Interpretant of the Percept, and of which the
Percept is the Dynamical Object, and is with some
considerable difficulty (as the history of psychology
shows), distinguished from the Immediate Object, though
the distinction is highly significant.(*)
But not to interrupt our train of thought let us go on
to note that while the Immediate Object of a Percept is
excessively vague, yet natural thought makes up for that
lack (as it almost amounts to), as follows. A late
Dynamical Interpretant of the whole complex of Percepts
is the Seme of a Perceptual Universe that is represented
in instinctive thought as determining the original
Immediate Object of every Percept.(**)
Of course, I must be understood as talking not
psychology, but the logic of mental operations.
Subsequent Interpretants furnish new Semes of Universes
resulting from various adjunctions to the Perceptual
Universe. They are, however, all of them, Interpretants
of Percepts. Finally, and in
particular, we get a Seme of that highest of all
Universes which is regarded as the Object of every true
proposition, and which, if we name it [at] all, we call
by the somewhat misleading title of "The Truth." |
| |
|
|
| 540. |
That said, let
us go back and ask this question: How is it that the
Percept, which is a Seme, has for its direct Dynamical
Interpretant the Perceptual Judgment, which is a Pheme?
For that is not the usual way with Semes, certainly. All
the examples that happen to occur to me at this moment
of such action of Semes are instances of Percepts,
though doubtless there are others. Since not all
Percepts act with equal energy in this way, the
instances may be none the less instructive for being
Percepts. However, Reader, I beg you will think this
matter out for yourself, and then you can see --I wish I
could--whether your independently formed opinion does
not fall in with mine. My opinion is that a pure
perceptual Icon--and many really great psychologists
have evidently thought that Perception is a passing of
images before the mind's eye, much as if one were
walking through a picture gallery - could not have a
Pheme for its direct Dynamical Interpretant. I desire,
for more than one reason, to tell you why I think so,
although that you should today appreciate my reasons
seems to be out of the question. Still, I wish you to
understand me so far as to know that, mistaken though I
be, I am not so sunk in
ntellectual night as to be dealing lightly with
philosophic truth when I aver that weighty reasons have
moved me to the adoption of my opinion; and I am also
anxious that it should be understood that those reasons
have not been psychological at all but are purely
logical. My reason, then, briefly stated and abridged is
that it would be illogical for a pure Icon to have a
Pheme for its Interpretant, and I hold it to be
impossible for thought not subject to self-control, as a
Perceptual Judgment manifestly is not, to be illogical.
I dare say this reason may excite your derision or
disgust, or both; and if it does I think none the worse
of your intelligence. You prob- ably opine, in the first
place, that there is no meaning in saying that thought
which draws no Conclusion is illogical, and that, at any
rate there is no standard by which I can judge whether
such thought is logical or not; and in the second place,
you probably think that if self-control has any
essential and important relation to logic which 1 guess
you either deny or strongly doubt it can only be that it
is that which makes thought Logical or else which
establishes the distinction between the logical and the
illogical, and that in any event it has to be such as it
is and would be logical, or illogical, or bath, or
neither whatever course it should take. But though an
Interpretant is not necessarily a Conclusion, yet a
Conclusion is necessarily an
Interpretant. So that if an Interpretant is not subject
to the rules of Conclusions there is nothing monstrous
in my thinking it is subject to some generalization of
such rules. For any evolution of thought, whether it
leads to a Conclusion or not, there is a certain normal
course, which is to be determined by considerations not
in the least psychological, and which I wish to expound
in my next article;(*)
and while I entirely agree, in opposition to
distinguished logicians, that normality can be no
criterion for what I call rationalistic reasoning, such
as alone is admissible in science, yet it is precisely
the criterion of instinctive or common-sense reasoning
which, within its own field, is much more trustworthy
than rationalistic reasoning. In my opinion, it is
self-control which makes any other than the normal
course of thought possible just as nothing else makes
any other than the normal course of action possible; and
just as it is precisely that that gives room for an
ought-to-be of conduct, I mean Morality, so it equally
gives room for an ought-to-be of thought, which is Right
Reason; and where there is no self-control, nothing but
the normal is possible. If your reflections have led you
to a different conclusion from mine, I can still hope
that when you come to read my next article, in which I
shall endeavor to show what the forms of thought are, in
general and in some detail you may yet find that I have
not missed the truth. |
| |
|
|
|
541. |
But supposing
that I am right, as I probably shall be in the opinions
of some readers, how then is the Perceptual Judgment to
be explained? In reply, I note that a Percept cannot be
dismissed at will, even from memory. Much less can a
person prevent himself from perceiving that which as we
say, stares him in the face. Moreover, the evidence is
overwhelming that the perceiver is aware of this
compulsion upon him; and if I cannot say for certain how
this knowledge comes to him, it is not that I cannot
conceive how it could come to him, but that, there being
several ways in which this might happen, it is difficult
to say which of those ways actually is followed. But
that discussion belongs to psychology; and I will not
enter upon it. Suffice it to say that the perceiver
explaining Abduction,(n1)
the attribution of Existence to it in the Perceptual
Judgment is virtually and in an extended sense, a
logical Abductive Inference nearly approximating to
necessary inference. But my next paper will throw a
flood of light upon the logical affiliation of the
Proposition, and the Pheme generally, to coercion. |
| |
|
|
|
542. |
That conception of Aristotle which is
embodied for us in the cognate origin of the terms
actuality and activity is one of the most deeply
illuminating products of Greek thinking. Activity
implies a generalization of effort; and effort is a two-
sided idea, effort and resistance being inseparable, and
there- fore the idea of Actuality has also a dyadic
form. |
| |
|
|
|
543. |
No cognition
and no Sign is absolutely precise, not even a Percept;
and indefiniteness is of two kinds, indefiniteness as to
what is the Object of the Sign, and indefiniteness as to
its Interpretant, or indefiniteness in Breadth and in
Depth.(*)
Indefiniteness in Breadth may be either Implicit or
Explicit. What this means is best conveyed in an
example. The word donation is indefinite as to who makes
the gift, what he gives, and to whom he gives it. But it
calls no attention, itself, to this indefiniteness. The
word gives refers to the same sort of fact, but its
meaning is such that that meaning is felt to be
incomplete unless those items are, at least formally,
specified; as they are in "Somebody gives something to
some person (real or artificial)." An ordinary
Proposition(**)
ingeniously contrives to convey novel information
through Signs whose significance depends entirely on the
interpreter's familiarity with them; and this it does by
means of a "Predicate," i.e., a term explicitly
indefinite in breadth, and defining its breadth by means
of "Subjects," or terms whose breadths are somewhat
definite, but whose informative depth (i.e., all the
depth except an essential superficies) is indefinite,
while conversely the depth of the Subjects is in a
measure defined by the Predicate. A Predicate is either
non-relative, or a monad, that is, is explicitly
indefinite in one extensive respect, as is "black"; or t
is a dyadic relative, or dyad, such as "kills," or it is
a polyadic relative, such as "gives." These things must
be diagrammatized in our system. Something more needs to
be added under the same head. you will observe that
under the term "Subject" I include, not only the subject
nominative, but also what the grammarians call the
direct and the indirect object, together, in some cases,
with nouns governed by prepositions. Yet there is a
sense in which we can continue to say that a Proposition
has but one Subject for example, in the proposition,
"Napoleon ceded Louisiana to the United States," we may
regard as the Subject the ordered triplet
"Napoleon--Louisiana--the United States" and as the
Predicate, "has for its first member, the agent or party
of the first part, for its second member the object and
for its third member the party of the second part of one
and the same act of cession." The view that there are
three subjects is, however, preferable for most
purposes, in view of its being so much more analytical,
as will soon appear. |
| |
|
|
|
544. |
All general or
definable Words, whether in the sense of Types or of
Tokens, are certainly Symbols. That is to say, they
denote the objects that they do by virtue on y o there
being a habit that associates their signification with
them. As to Proper Names, there might perhaps be a
difference of opinion especially if the Tokens are
meant. But they should probably be regarded as Indices,
since the actual connection (as we listen to talk), of
Instances of the same typical words with the same
Objects, alone causes them to be interpreted as denoting
those Objects. Excepting, if necessary, propositions in
which all the subjects are such signs as these, no
proposition can be expressed without the use of Indices.(n1)
If, for example, a man remarks, "Why, it is raining!" it
is only by some such circumstances as that he is now
standing here looking out at a window as he speaks,
which would serve as an Index (not,
however, as a Symbol) that he is speaking of this place
at this time, whereby we can be assured that he cannot
be speaking of the weather on the satellite of Procyon,
fifty centuries ago. Nor are Symbols and Indices
together generally enough. The arrangement of the words
in the sentence, for instance, must serve as Icons, in
order that the sentence may be understood. The chief
need for the Icons is in order to show the Forms of the
synthesis of the elements of thought. For in precision
of speech, Icons can represent nothing but Forms and
Feelings. That is why Diagrams are indispensable in all
Mathematics, from Vulgar Arithmetic up, and in Logic are
almost so. For Reasoning, nay, Logic generally, hinges
entirely on Forms. you Reader, will not need to be told
that a regularly stated Syllogism is a Diagram; and if
you take at random a half dozen out of the hundred odd
logicians who plume themselves upon not belonging to the
sect of Formal Logic, and if from this latter sect you
take another half dozen at random, you will find that in
proportion as the former avoid diagram.s, they utilize
the syntactical Form of their sentences. No pure Icons
represent anything but Forms; no pure Forms are
represented by anything but Icons. As for Indices, their
utility especially shines where other Signs fail.
Extreme precision being desired in the description of a
red color, should I call it vermillion, I may be
criticized on the ground that vermillion differently
prepared has quite different hues, and thus I may be
driven to the use of the color-wheel, when I shall have
to Indicate four disks individually, or I may say in
what proportions light of a given wave-length is to be
mixed with white light to produce the color I mean. The
wave-length being stated in fractions of a micron, or
millionth of a meter, is referred through an Index to
two lines on an individual bar in the Pavillon de
Breteuil, at a given temperature and under a pressure
measured against gravity at a certain station and
(strictly) at : given date, while the mixture with
white, after white has beer fixed by an Index of an
individual light, will require at least one new Index.
But of superior importance in Logic is the use of
Indices to denote Categories and Universes,(n2)
which are classes that, being enormously large, very
promiscuous, and known but in small part, cannot be
satisfactorily defined, and therefore can only be
denoted by Indices. Such, to give but a single instance,
is the collection of all things in the Physical
Universe. If anybody, your little son for example, who
is such an assiduous researcher, always asking, What is
the Truth (ti estin aleqeia); but like "jesting
Pilate," will not always stay for an answer, should ask
you what the Universe of things physical is, you may, if
convenient, take him to the Rigi-Kulm, and about sunset,
point out all that is to be seen of Mountains, Forests,
Lakes, Castles, Towns, and then, as the stars come out,
all there is to be seen in the heavens, and all that
though not seen, is reasonably conjectured to be there;
and then tell him, "Imagine that what is to be seen in a
city back yard Lo grow to all you can see here, and then
let this grow in the same proportion as many times as
there are trees in sight from here, and what you would
finally have would be harder to find in the Universe
than the finest needle in America's yearly crop of hay."
But such methods are perfectly futile: Universes cannot
be described. |
| |
|
|
|
545. |
Oh, I overhear
what you are saying, 0 Reader: that a Universe and a
Category are not at all the same thing; a Universe being
a receptacle or class of Subjects, and a Category being
a mode of Predication, or class of Predicates. I never
said they were the same thing; but whether you describe
the two correctly is a question for careful study |
| |
|
|
|
546. |
Let us begin
with the question of Universes. It is rather a question
of an advisable point of view than of the truth of a
doctrine. A logical universe is, no doubt, a collection
of logical subjects, but not necessarily of
meta-physical Subjects, or "substances"; for it may be
composed of characters, of elementary facts, etc. See my
definition in Baldwin's Dictionary.(*)
Let us first try whether we may not assume that there is
but one kind of Subjects which are either existing
things or else quite fictitious. Let it be asserted that
there is some married woman who will commit suicide in
case her husband fails in business. Surely that is a
very different proposition from the
assertion that some married woman will commit suicide if
all married men fail in business. Yet if nothing is real
but existing things, then, since in the former
proposition nothing whatever is said as to what the lady
will or will not do if her husband does not fail in
business, and since of a given married couple this can
only be false if the fact is contrary to the assertion,
it follows it can only be false if the husband does fail
in business and if the wife then fails to commit
suicide. But the proposition only says that there is
some married couple of which the wife is of that temper.
Consequently, there are only two ways in which the
proposition can be false, namely, first, by there not
being any married couple, and secondly, by every married
man failing in business while no married woman commits
suicide. Consequently, all that is required to make the
proposition true is that there should either be some
married man who does not fail in business, or else some
married woman who commits suicide. That is, the
proposition amounts merely to asserting that there is a
married woman who will commit suicide if every married
man fails in business. The equivalence of these two
propositions is the absurd result of admitting no
reality but existence. If, however, we suppose that to
say that a woman will suicide if her husband fails,
means that every possible course of events would either
be one in which the husband would not fail or one in
which the wife would commit suicide, then, to make that
false it will not be requisite for the husband actually
to fail, but it will suffice that there are possible
circumstances under which he would fail, while yet his
wife would not commit suicide. Now you will observe that
there is a great difference between the two following
propositions:
First, There is some
one married woman who under all possible conditions
would commit suicide or else her husband would not
have failed.
Second, Under all
possible circumstances there is some married woman
or other who would commit suicide, or else her
husband would not nave failed.
The former of these is what is
really meant by saying that there is some married woman
who would commit suicide if her husband were to fail,
while the latter is what the denial of any possible
circumstances except those that really take place
logically leads to [our] interpreting (or virtually
interpreting), the Proposition as asserting. |
| |
|
|
|
547. |
In other
places,(*)
I have given many other reasons for my firm belief that
there are real possibilities. I also think, however,
that, in addition to actuality and possibility, a third
mode of reality must be recognized in that which, as the
gipsy fortune-tellers express it, is "sure to come
true," or, as we may say is destined,(n1)
although I do not mean to assert that this is
affirmation rather than the negation of this Mode of
Reality. I do not see by what confusion of thought
anybody can persuade himself that he does not believe
that tomorrow is destined to come. The point is that it
is today really true that to-morrow the sun will rise;
or that, even if it does not, the clocks or something,
will go on. For if it be not real it can only be
fiction: a Proposition is either True or False. But we
are too apt to confound destiny with the impossibility
of the opposite. I see no impossibility in the sudden
stoppage of everything. In order to show the difference,
I remind you that "impossibility" is that which, for
example, describes the mode of falsity of the idea that
there should be a collection of objects so multitudinous
that there would not be characters enough in the
universe of characters to distinguish all those things
from one another. Is there anything of that sort about
the stoppage of all motion? There is, perhaps, a Law of
nature against it; but that is all. However, J will
postpone the consideration of that point. Let us, at
least, provide for such a mode of being in our system of
diagrammatization, since it may turn out to be needed
and, as I think, surely will. |
| |
|
|
|
548. |
I will proceed
to explain why, although I am not prepared to deny that
every proposition can be represented, and that I must
say, for the most part very conveniently, under your
view that the Universes are receptacles of the Subjects
alone, I, nevertheless, cannot deem that mode of
analyzing Propositions to be satisfactory. And to
begin with, I trust you will all agree with me that no
analysis, whether in logic, in chemistry, or in any
other science, is satisfactory, unless it be thorough,
that is, unless it separates the compound into
components each entirely homogeneous in itself, and
therefore free from the smallest admixture of any of the
others. It follows that in the Proposition "Some Jew is
shrewd," the Predicate is "Jew-that-is-shrewd" and the
Subject is Something, while in the proposition "Every
Christian is meek," the Predicate is "Either not
Christian or else meek," while the Subject is Anything;
unless, indeed we find reason to prefer to say that this
Proposition means "It is false to say that a person is
Christian of whom it is false to say that he is meek."
In this last mode of analysis, When a Singular Subject
is not in question (which case will be examined later),
the only Subject is Something. Either of these two modes
of analysis [differentiates] quite [clearly] the Subject
from any Predicative ingredients; and at first sight,
either seems quite favorable to this view that it is
only the Subjects which this to the Universes. Let us,
however, consider the following two forms of
propositions:
A(*)
Any adept alchemist could produce a
philosopher's stone of some kind or other,
B There is one kind of philosopher's stone that
any adept alchemist could produce.
We can express these in the principle that the
Universes are receptacles of Subjects as follows:
A1 The Interpreter having selected any individual
he likes, and called it A, an object B can be found,
such that, Either A would not be an adept alchemist,
or B would be a philosopher's stone of some kind,
and A could produce B.
B1 Something, B, might be found, such that, no
matter what the Interpreter might select and call A,
B would be a philosopher's stone of some kind, while
either A would not be an adept alchemist, or else A
could produce B.
In these forms there are two Universes, the one of
individuals selected at pleasure by the interpreter of
the proposition, the other of suitable objects.
I will now express the same two propositions on the
principle that each Universe consists, not of Subjects,
but the one of True assertions, the other of False, but
each to the effect that there is something of a given
description.
1. This is false: That something, P, is an adept
alchemist and that this is false, that while
something, S, is a philosopher's stone of some kind,
P could produce S.
2. This is true: That something, S, is a
philosopher's stone of some kind; and this is false,
that something, P is an adept alchemist while this
is false, that P could produce S.
Here, the whole proposition is mostly made up of the
truth or falsity of assertions that a thing of this or
that description exists, the only conjunction being
"and." That this method is highly analytic is manifest.
Now since our whole intention is to produce a method for
the perfect analysis of propositions the superiority of
this method over the other for our purpose is
undeniable. Moreover, in order to illustrate how that
other might lead to false logic, I will tack the
predicate of B1 in its objectionable form, upon the
subject of A1 in the same form and vice versa. I shall
thus obtain two propositions which that method
represents as being as simple as are Nos I and 2 We
shall see whether they are so Here they are:(**)
3. The Interpreter, having designated any object
to be called A, an object B may be found such that B
is a philosopher's stone of some kind while either A
is not an adept alchemist or else A could produce B
4. Something, B, may be found such that no matter
what the interpreter may select, and call A, Either
A would not be an adept alchemist, or g would be a
philosopher's stone of some kind, and A could
produce B.
Proposition 3 may be expressed in ordinary language
thus: There is a kind of philosopher's stone, and if
there be any adept alchemist, he could produce a
philosopher's stone of some kind. That is, No. 3 differs
from A, A1 and I only in adding that there is a kind of
philosopher's stone. It differs from B B1 and 2 in not
saying that any two adepts could produce the same kind
of stone (nor that any adept could produce any existing
kind); while B, BI and 2 assert that some kind is both
existent and could be made by every adept.
Proposition 4, in ordinary language is: If there
be (or were) an adept alchemist, there is (or would be)
a kind of philosopher's stone that any adept could
produce This asserts the substance of B, B1 and 2, but
only conditionally upon the existence of an adept; but
it asserts what A A and 1 do not, that all adepts could
produce some one kind of stone and this is precisely the
difference between No. 4 and A1
To me it seems plain that the propositions 3 and 4
are both less simple than No. 1
and less simple than No 2 each adding some thing to one
of the pair first given and asserting the other
conditionally. Yet the method of treating the Universes
as receptacles for the metaphysical Subjects only
involves as a consequence the representation of 3 and 4
as quite on a par with 1 and 2.
It remains to show that the other method does not
carry this error with it. [If] it is the states of
things affirmed or denied that are contained in the
universes, then the propositions [3 and 4] become as
follows:
5. This is true: that there is a philosopher's
stone of some kind, S, and that it is false that
there is an adept, A, and that it is false that A
could produce a philosopher's stone of some kind,
S'. (Where it is neither asserted nor denied that S
and S' are the same, thus distinguishing this from
2.)
6. This is false: That there is an adept, A, and
that this is false: That there is a stone of a kind,
S, and this is false: That there is an adept, A',
and that this is false: That A' could produce a
stone of the kind S. (Where again it is neither
asserted nor denied that A and A1 are identical, but
the point is that this proposition holds even if
they are not identical, thus distinguishing this
from 1.)
These forms exhibit the greater complexity of
Propositions 3 and 4, by showing that they really relate
to three individuals each; that is to say, 3 to two
possible different kinds of stone as
well as to an adept; and 4 to two possible different
adepts, and to a kind of stone. Indeed, the two forms 3
and 4(***)
are absolutely identical in meaning with the following
different forms on the same theory. Now it is, to say
the least, a serious fault in a method of analysis that
it can yield two analyses so different of one and the
same compound.
7. An object, B, can be found,
such that whatever object the interpreter may select
and call A, an object, B, can thereupon be found
such that B is an existing kind of philosopher's
stone, and either A would not be an adept or else B'
is a kind of philosopher's stone such as A could
produce.
8. Whatever individual the
Interpreter may choose to call A, an object, B, may
be found, such that whatever individual the
Interpreter may choose to call A', Either A is not
an adept or B is an existing kind of philosopher's
stone, and either A1 is not an adept or else A1
could produce a stone of the kind B.
But while my forms are perfectly
analytic, the need of diagrams to exhibit their meaning
to the eye (better than merely giving a separate line to
every proposition said to be false) is painfully
obtrusive. (n1) |
| |
|
|
|
549. |
I will now say a few words about what
you have called Categories but for which I prefer the
designation Predicaments and which you have explained as
predicates of predicates. That wonderful operation of
hypostatic abstraction by which we seem to create entia
rationis that are, nevertheless, sometimes real,
furnishes us the means of turning predicates from being
signs that we think or think through, into being
subjects thought of. We thus think of the thought-sign
itself, making it the object of another thought-sign.
Thereupon, we can repeat the operation of hypostatic
abstraction, and from these second intentions derive
third intentions. Does this series proceed endlessly? I
think not. What then are the characters of its different
members? My thoughts on this subject are not yet
harvested. I will only say that the subject concerns
Logic, but that the divisions so obtained must not be
confounded with the different Modes of Being:(*)
Actuality, Possibility, Destiny (or Freedom from
Destiny). On the contrary, the succession of Predicates
of Predicates is different in the different Modes of
Being. Meantime, it will be proper that in our system of
diagrammatization we should provide for the division,
whenever needed, of each of our three Universes of modes
of reality into Realms for the different Predicaments. |
| |
|
|
| 550. |
All the
various meanings of the word "Mind," Logical
Metaphysical, and Psychological, are apt to be
confounded more or less, partly because considerable
logical acumen is required to distinguish some of them,
and because of the lack of any machinery to support the
thought in doing so, partly because they are so many,
and partly because (owing to these causes) they are all
called by one word, "Mind." In one of the narrowest and
most concrete of its logical meanings, a Mind is that
Seme of The Truth, whose determinations become Immediate
Interpretants of all other Signs whose Dynamical
Interpretants are dynamically connected.(*)
In our Diagram the same thing which represents The Truth
must be regarded as in another way representing the
Mind, and indeed, as being the Quasi-mind of all the
Signs represented on the Diagram. For any set of Signs
which are so connected that a complex of two of them can
have one interpretant, must be Determinations of one
Sign which is a Quasi-mind. |
| |
|
|
|
551. |
Thought is not
necessarily connected with a brain. It appears in the
work of bees, of crystals, and throughout the purely
physical world; and one can no more deny that it is
really there, than that the colors, the shapes, etc., of
objects are really there. Consistently adhere to that
unwarrantable denial, and you will be driven to some
form of idealistic nominalism akin to Fichte's. Not only
is thought in the organic world, but it develops there.
But as there cannot be a General without Instances
embodying it, so there cannot be thought
without Signs. We must here give " Sign " a very wide
sense, no doubt, but not too wide a sense to come within
our definition, Admitting that connected Signs must have
a Quasi-mind, ,t may further be declared that there can
be no isolated sign. Moreover, signs require at least
two Quasi-minds; a Quasi- utterer and a
Quasi-interpreter; and although these two are at one
(i.e., are one mind) in the sign itself, they must
nevertheless be distinct. In the Sign they are, so to
say, welded. Accordingly, it is not merely a fact of
human Psychology, but a necessity of Logic, that every
logical evolution of thought should be dialogic. You may
say that all this is loose talk; and 1 admit that, as it
stands, it has a large infusion of arbitrariness. It
might be filled out with argument so as to remove the
greater part of this fault; but in the first place, such
an expansion would require a volume -- and an uninviting
one; and in the second place, what I nave been saying is
only to be applied to a slight determination of our
system of diagrammatization, which it will only slightly
affect; so that, should it be incorrect, the utmost
certain effect will be a danger that our system may not
represent every variety of non-human thought. |
| |
|
|
| |
5. TINCTURED EXISTENTIAL GRAPHS |
|
552. |
There now
seems to remain no reason why we should not proceed
forthwith to formulate and agree upon |
| |
|
| |
THE
CONVENTIONS |
| |
|
| |
DETERMINING
THE FORMS AND INTERPRETATIONS OF
EXISTENTIAL GRAPHS |
| |
|
| |
Convention
the First: Of the Agency of the Scripture. We are to
imagine that two parties(n1)
collaborate in composing a Pheme and in operating upon
this so as to develop a Delome. (Pro- vision shall be
made in these Conventions for expressing every kind of
Pheme as a Graph;
(n2) and it
is certain that the Method could be applied to aid the
development and analysis of any kind of purposive
thought. But hitherto no Graphs have been
studied but such as are Propositions; so that, in the
resulting uncertainty as to what modifications of the
Conventions might be required for other applications,
they have mostly been here stated as if they were only
applicable to the expression of Phemes and the working
out of necessary conclusions.)
The two collaborating parties shall
be called the Graphist and the Interpreter. The Graphist
shall responsibly scribe each original Graph and each
addition to it, with the proper indications of the
Modality to be attached to it, the relative Quality(n3)
of its position, and every particular of its dependence
on and connections with other graphs. The Interpreter is
to make such erasures and insertions of the Graph
delivered to him by the Graphist as may accord with the
"General Permissions" deducible from the Conventions and
with his own purposes. |
| |
|
|
|
553. |
Convention
the Second; Of the Matter of the Scripture and the
Modality(n1)
of the Phemes expressed. The matter which the
Graph-instances are to determine, and which thereby
becomes the Quasi-mind in which the Graphist and
Interpreter are at one, being a Seme of The Truth,
(n2) that is,
of the widest Universe of Reality,
and at the same time, a Pheme of all that is tacitly
taken for granted between the Graphist and Interpreter
from the outset of their discussion, shall be a sheet
called the Phemic Sheet, upon which signs can be scribed
and from which any that are already scribed in any
manner (even though they be incised) can be erased. But
certain parts of other sheets not having the
significance of the Phemic |
| |
 |
| |
Figure 197 |
| |
sheet, but on
which Graphs can be scribed and erased, shall be
sometimes inserted in the Phemic sheet and exposed to
view, as the Third Convention shall show. Every part of
the exposed surface shall be tinctured in one or another
of twelve tinctures. These are divided into three
classes of four tinctures each, the class-characters
being called Modes of Tincture, or severally, Color,
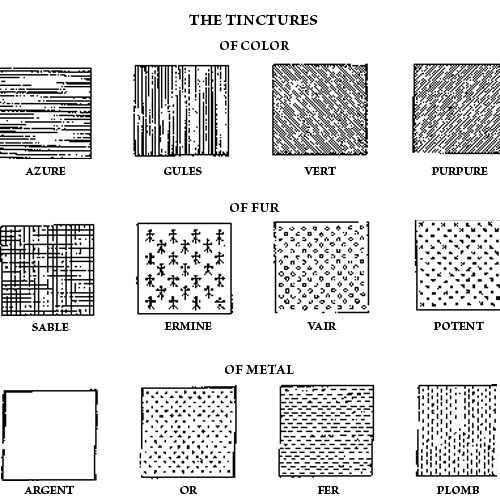
Fur, and Metal. The tinctures of Color are Azure,
Gules, Vert, and Purpure. Those of Fur are Sable,
Ermine, Vair, and Potent. Those of Metal are Argent, Or,
Fer, and Plomb. The Tinctures will in practice be
represented as in Fig. 197.(n3)
The whole of any continuous part of the exposed
surface in one tincture shall be termed a Province. The
border of the sheet has one tincture all round; and we
may imagine that it was chosen from among twelve, in
agreement between the Graphist and the Interpreter at
the outset. The province of the border may be called the
March. Provinces adjacent to the March are to be
regarded as overlying it. Provinces adjacent to those
Provinces, but not to the March, are to be regarded as
overlying the provinces adjacent to the March, and so
on. We are to imagine that the Graphist always finds
Provinces where he needs them. |
| |
|
|
| 554. |
When any
representation of a state of things consisting in the
applicability of a given description to an individual or
limited set of individuals otherwise indesignate is
scribed, the Mode of Tincture of the province on which
it is scribed shows whether the Mode of Being which is
to be affirmatively or negatively attributed to the
state of things described is to be that of Possibility,
when Color will be used; or that of Intention, indicated
by Fur; or that of Actuality shown by Metal. Special
understandings may determine special tinctures to refer
to special varieties of the three genera of Modality.
Finally, the Mode of Tincture of the March may determine
whether the Entire Graph is to be understood as
interrogative, Imperative, or Indicative. |
| |
|
|
| 555. |
Convention
the Third: Of Areas enclosed within, but severed from,
the Phemic Sheet. The Phemic Sheet is to be imagined
as lying on the smoother of the two surfaces or sides of
a Leaf, this side being called the recto, and to consist
of so much of this side as is continuous with the March.
Other parts of the recto may be exposed to view. Every
Graph-instance on the Phemic Sheet is posited
unconditionally (unless, according to an agreement
between Graphist and Interpreter, the Tincture of its
own Province or of the March should indicate a
condition) and every Graph-instance on the recto is
posited affirmatively and, in so far as it is
indeterminate, indefinitely. |
| |
|
|
| 556. |
Should the
Graphist desire to negative a Graph, he must scribe it
on the verso, and then, before delivery to the
Interpreter, must make an incision, called a Cut,
through the Sheet all the way round the Graph-instance
to be denied, and must then turn over the excised piece,
so as to expose its rougher surface carrying the
negatived Graph-instance. This reversal of the piece is
to be conceived to be an inseparable part of the
operation of making a Cut.(n1)
But if the Graph to be negatived includes a Cut the
twice negatived Graph within that Cut must be scribed on
the recto, and so forth. The part of the exposed surface
that is continuous with the part just outside the Cut is
called the Place of the Cut. A Cut is neither a Graph
nor a Graph-instance; but the Cut, together with all
that it encloses exposed is termed an Enclosure, and is
conceived to be an Instance of a Graph scribed on the
Place of the Cut, which is also termed the Place of the
Enclosure. The surface within the Cut, continuous with
the parts just within it, is termed the Area of the Cut
and of the Enclosure; and the part of the recto
continuous with the March (i.e., the Phemic Sheet) is
likewise termed an Area, namely the Area of the Border
The Copulate of all that is scribed on any one Area,
including the Graphs of which the Enclosures whose Place
is this Area are Instances, is called the Entire Graph
of that Area; and any part of the Entire Graph, whether
graphically connected with or disconnected from the
other parts, provided it might be the
Entire Graph of the Sheet, is termed a Partial Graph of
the Area. |
| |
|
|
| 557. |
There may be
any number of Cuts, one within another, the Area of one
being the Place of the next, and since the Area of each
is on the side of the leaf opposite to its Place, it
follows that recto Areas may be exposed which are not
parts of the Phemic Sheet. Every Graph-instance on a
recto Area is affirmatively posited, but is posited
conditionally upon what- ever may be signified by the
Graph on the Place of the Cut of which this Area is the
Area. (It follows that Graphs on Areas of different
Enclosures on a verso Place are only alternately
affirmed, and that while only the Entire Graph of the
Area of an Enclosure on a recto Place is denied, but not
its different Partial Graphs, except alteratively, the
Entire Graphs of Areas of different Enclosures on one
recto Place are copulatively denied.) |
| |
|
|
| 558. |
Every
Graph-instance must lie upon one Area
(n1) although
an Enclosure may be a part of it. Graph-instances on
different Areas are not to be considered as, nor by any
permissible latitude of speech to be called, Parts of
one Graph-instance, nor Instances of Parts of one Graph;
for it is only Graph-instances on one Area that are
called Parts of one Graph-instance, and that only of a
Graph-instance on that same Area; for though the Entire
Graph on the Area of an enclosure is termed the Graph of
the Enclosure, it is no Part of the Enclosure and is
connected with it only through a denial. |
| |
|
|
| 559. |
Convention
the Fourth: Concerning Signs of Individuals and of
Individual Identity. A single dot, not too minute,
or single congeries of contiguous pretty large dots,
whether in the form of a line or surface, when placed on
any exposed Area, will refer to a single member of the
Universe to which the Tincture of that Area refers, but
will not thereby be made to refer determinately to any
one. But do not forget that separate
dots, or separate aggregates of dots, will not
necessarily denote different Objects. |
| |
|
|
| 560. |
By a rheme, or
predicate, will here be meant a blank form of
proposition which might have resulted by striking out
certain parts of a proposition, and leaving a blank in
the place of each, the parts stricken out being such
that if each blank were filled with a proper name, a
proposition (however nonsensical) would thereby be
recomposed. An ordinary predicate of which no analysis
is intended to be represented will usually be written in
abbreviated form, but having a particular point an the
periphery of the written form appropriated to each of
the blanks that might be filled with a proper name. Such
written form with the appropriated points shall be
termed a Spot - and each appropriated point of its
periphery shall be called a peg of the Spat. If a heavy
dot is placed at each Peg the Spot will became a Graph
expressing a proposition in which every blank is filled
by a ward (or concept denoting an indefinite individual
abject, "something." |
| |
|
|
| 561. |
A heavy line
shall be considered as a continuum of contiguous dots;
and since contiguous dots denote a single individual,
such a line without any point of branching will signify
the identity of the individuals denoted by its
extremities, and the type of such unbranching line shall
be the Graph of Identity, any instance of which (on one
area, as every Graph-instance must be) shall be called a
Line of Identity. The type of a three-way point of such
a line (Fig. 198) shall be the Graph of Teridentity; and
it shall be considered as composed of three contiguous
Pegs of a Spot of Identity. An extremity |
| |
 |
 |
|
Figure 198 |
Figure 199 |
|
| |
of a Line of
Identity not abutting upon another such Line in another
area shall be called a Loose End. A heavy line, whether
confined to one area or not (and therefore not generally
being a Graph-instance) of which two extremities abut
upon pegs of spots shall be called a Ligature. Two lines
cannot abut upon the same peg other
than a point of teridentity. (The purpose of this rule
is to force the recognition of the demonstrable logical
truth that the concept of teridentity is not mere
identity. It is identity and identity, but this "and" is
a distinct concept, and is precisely that of
teridentity) A Ligature crossing a Cut is to be
interpreted as unchanged in meaning by erasing the part
that crosses to the Cut and attaching to the two Loose
Ends so produced two Instances of a Proper Name nowhere
else used; such a Proper name (for which a capital
letter will serve) being termed a Selective.(n1)
In the interpretation of Selectives it is often
necessary to observe the rule which holds throughout the
System, that the Interpretation of Existential Graphs
must be endoporeutic that is the application of a Graph
on the Area of a Cut will depend on the predetermination
of the application of that which is on the Place of the
Cut.
In order to avoid the intersection of Lines of Identity
either a Selective may be employed, or a Bridge, which
is imagined to be a bit of paper ribbon, but will in
practice be pictured as in Fig. 199. |
| |
|
|
| 562. |
Convention the
Fifth: Of the Connections of Graph Instances. Two
partial Graph-Instances are said to be individually and
directly connected, if, and only if, in the Entire
Graph, one individual is, either unconditionally or
under some condition, and whether affirmatively or
negatively, made a Subject of both. Two Graph-Instances
connected by a ligature are explicitly and definitely
individually and directly connected. Two Graph-Instances
in the same Province are thereby explicitly, although
indefinitely, individually and directly connected, since
both, or one and the negative of the other, or the
negative of both, are asserted to be true or false
together, that is, under the same circumstances,
although these circumstances are not formally defined,
but are left to be interpreted according to the nature
of the case. Two Graph-Instances not in the same
Province, though on the same Mode of Tincture, are
only in so far connected that both are in the same
Universe. Two Graph-Instances in different Modes of
Tincture are only in so far connected that both, or one
and the negative of the other, or the negative of both,
are posited as appertaining to the Truth. They cannot be
said to have any individual and direct connection. Two
Graph-Instances that are not individually connected
within the innermost Cut which contains them both cannot
be so connected at all; and every ligature connecting
them is meaningless and may be made or broken. |
| |
|
|
| 563. |
Relations
which do not imply the occurrence in their several
universes of all their correlates must not be expressed
by Spots or single Graphs,(n1)
but all such relations can be expressed in the System. |
| |
|
|
| 564. |
I will now
proceed to give a few examples of Existential Graphs in
order to illustrate the method of interpretation, and
also the Permissions of Illative Transformation of them.
If you carefully examine the above conventions, you will
find that they are simply the development, and excepting
in their insignificant details, the inevitable result of
the development of the one convention that if any Graph,
A, asserts one state of things to be real and if another
graph, B, asserts the same of another state of things,
then AB, which results from setting both A and B upon
the sheet, shall assert that both states of things are
real. This was not the case with my first system of
Graphs, described in Vol. VII of The Monist,(*)
which I now call Entitative Graphs. But I was forced to
this principle by a series of considerations which
ultimately arrayed them- selves into an exact logical
deduction of all the features of Existential Graphs
which do not involve the Tinctures. I nave no room for
this here; but I state some of the points arrived at
somewhat in the order in which they first presented
themselves.
In the first place, the most perfectly analytical
system of representing propositions must enable us to
separate illative transformations into indecomposable
parts Hence an illative transformation from any
proposition, A, to any other B must
in such a system consist in first transforming A into
AB, followed by the transformation of AB into B. For an
omission and an insertion appear to be indecomposable
transformations and the only indecomposable
transformations. That is, if A can be transformed by
insertion into AB, and AB by omission in B, the
transformation of A into B can be decomposed into an
insertion and an omission. Accordingly, since logic has
primarily in view argument, and since the conclusiveness
of an argument can never be weakened by adding to the
premisses nor by subtracting from the conclusion, I
thought I ought to take the general form of argument as
the basal form of composition of signs in my
diagrammatization; and this necessarily took the form of
a "scroll," that is (see figs. 200, 201, 202) a curved
line without contrary flexure and returning |
| |
 |
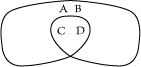 |
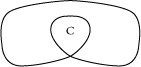 |
|
Figure 200 |
Figure 201 |
Figure 202 |
|
| |
into itself
after once crossing itself, and thus forming an outer
and an inner "close." I shall call the outer boundary
the Wall; and the inner, the Fence. In the outer I
scribed the Antecedent, in the inner the Consequent, of
a Conditional Proposition de inesse. The scroll
was not taken for this purpose at hap-hazard, but was
the result of experiments and reasonings by which 1 was
brought to see that it afforded the most faithful
Diagram of such a Proposition. This form once obtained,
the logically inevitable development brought me speedily
to the System of Existential Graphs. Namely, the idea of
the scroll was that fig. 200, for example, should assert
that if A be true (under the actual circumstances), then
C and D are both true. This justifies fig. 201, that if
both A and B are true, then both C and D are true, no
matter what B may assert, any insertion being permitted
in the outer close, and any omission from the inner
close. By applying the former clause of this rule to
fig. 202, we see that this scroll with the outer close
void, justifies the assertion that if no matter what
be true, C is in any case true; so that the two walls of
the scroll, when nothing is between them, fall together,
collapse, disappear, and leave only the contents of the
inner close standing, asserted, in the open field.
Supposing, then, that the contents of the inner scroll
had been CD, these would have been left standing, both
asserted; and we thus return to the principle that
writing assertions together on the open sheet asserts
them all. Now, Reader, if you will just take pencil and
paper and scribe the scroll expressing that if A be
true, then it is true that if B be true C and D are
true, and compare this with Fig. 201, which amounts to
the same thing in meaning, you will see that scroll
walls with a void between them collapse even when they
belong to different scrolls; and you will |
| |
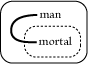 |
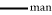 |
|
Figure 203 |
Figure 204 |
|
| |
further see
that a scroll is really nothing but one oval within
another. Since a Conditional de inesse (unlike other
conditionals) only asserts that either the antecedent is
false or the consequent is true, it all but follows that
if the latter alternative be suppressed by scribing
nothing but the antecedent, which may be any
proposition, in an oval, that antecedent is thereby
denied.(n1)
The use of a heavy line as a juncture signifying
identity is inevitable; and since Fig. 203 must mean
that if anything is a man, it is mortal, it will follow
that Fig. 204 must mean "Something is a man." |
| |
|
|
| 565. |
The first
permission of illative transformation is now evident as
follows: First Permission, called "The Rule of
Deletion and Insertion" Any Graph-Instance can be
deleted from any recto Area (including the severing of
any Line of Identity) and any Graph-Instance can be
inserted on any verso Area (including as a
Graph-Instance the juncture of any two Lines of Identity
of Points of Teridentity). |
| |
|
|
| 566. |
The justice of
the following will be seen instantly by students of any
form of Logical Algebra and with very little difficulty
by others: Second Permission, called "The Rule of
Iteration and Deiteration." Any Graph scribed on any
Area may be Iterated in or (if already Iterated) may be
Deiterated by a deletion from that Area or from any
other Area included within that. This involves the
Permission to distort a line of Identity at will.
To iterate a Graph means to scribe it again, while
joining by Ligatures every Peg of the new<xnstance to
the corresponding Peg of the Original Instance. To
deiterate a Graph is to erase a second Instance of it,
of which each Peg is joined by a Ligature to a first
Instance of it. One Area is said to be incLuded within
another if, and only if, it either is that Area or else
is the Area of a Cut whose Place is an Area which,
according to this definition, must be regarded as
incLuded within that other. By this Permission, Fig. 205
may be transformed into Fig.
206, and thence, by Permission No. 1, into Fig. 207. |
| |
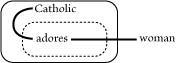 |
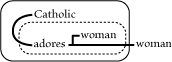 |
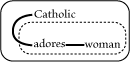 |
|
Figure 205 |
Figure 206 |
Figure 207 |
|
| |
|
|
| 567. |
We now come to
the Third Permission, which I shall state in a form
which is valid, sufficient for its purpose, and
convenient in practice, but which cannot be assumed as
an undeduced Permission, for the reason that it allows
us to regard the Inner close, after the Scroll is
removed, as being a part of the Area on which the Scroll
lies. Now this is not strictly either an Insertion or a
Deletion; and a perfectly analytical System of
Permissions should permit only the indecomposable
operations of Insertion and Deletion of Graphs that are
simple in expression. The more
scientific way would be to substitute for the Second and
Third Permissions the following Permission:
If an Area, Y, and an Area, 0, be
related in any of these four ways, viz., (l) If Y and 0
are the same Area; (2) If 0 is the Area of an Enclosure
whose Place is Y; (3) if 0 is the Area of an Enclosure
whose Place is the Area of a second Enclosure whose
Place is Y; or (4) if 0 is the Place of an Enclosure
whose Area is vacant except that it is the Place of an
Enclosure whose Area is Y, and except that it may
contain ligatures, identifying Pegs in 0 with Pegs in Y;
then, if 0 be a recto area, any simple Graph already
scribed upon Y may be iterated upon 0; where if 0 be a
verso Area, any simple Graph already scribed upon Y and
iterated upon 0 may be deiterated by being deleted or
abolished from 0.
These two Rules (of Deletion and
Insertion, and of Iteration and Deiteration) are
substantially all the undeduced Permissions needed; the
others being either Consequences or Explanations of
these. Only, in order that this may be true, it is
necessary to assume that all indemonstrable implications
of the Blank have from the beginning been scribed upon
<distant parts of the Phemic Sheet, upon any part of
which they may, therefore, be iterated at will. I will
give no list of these implications, since it could serve
no other purpose than that of warning beginners that
necessary propositions not included therein were
deducible from the other permissions. I will simply
notice two principles the neglect of which might lead to
difficulties. One of these is that it is physically
impossible to delete or otherwise get rid of a Blank in
any Area that contains a Blank, whether alone or along
with other Graph-Instances. We may, however, assume that
there is one Graph, and only one, an Instance of which
entirely fills up an Area, without any Blank. The other
principle is that, since a Dot merely asserts that some
individual object exists, and is thus one of the
implications of the Blank, it may.be inserted in any
Area and since the Dot will signify the same thing
whatever its size it may be regarded as an Enclosure
whose Area is filled with an Instance of that sole Graph
that excludes the Blank. The Dot, then, denies that
Graph, which may, therefore be understood as the absurd
Graph, and its signification may be formulated as
"Whatever you please is true." The absurd Graph may also
take the form of an Enclosure with its Area entirely
Blank, or enclosing only some Instance of a Graph
implied in the Blank. These two principles will enable
the Graphist to thread his way through some
Transformations which might otherwise appear paradoxical
and absurd.
Third Permission, called "The
Rule of the Double Cut." Two Cuts, one within another,
with nothing between them, unless it be Ligatures
passing from outside the outer Cut to inside the inner
one, may be made or abolished on any Area. |
| |
|
|
| 568. |
Let us now
consider the Interpretation of such Ligatures. For that
purpose, I first note that the Entire Graph of any recto
Area is a wholly particular and affirmative Proposition
or Copulation of such Propositions. By "wholly
particular," I mean, having for every Subject an
indesignate individual. The Entire Graph of any verso
Area is a wholly universal negative proposition or a
disjunction of such propositions. The first time one
hears a proper name pronounced, it is but a name,
predicated, as one usually gathers, of an existent, or
at least historically existent, individual object, of
which, or of whom, one almost always gathers some
additional information. The next time one hears the
name, it is by so much the more definite; and almost
every time one hears the name one gains in familiarity
with the object. A Selective is a Proper Name met with
by the Interpreter for the first time. But it always
occurs twice, and usually on different areas. Now the
Interpretation, by Convention No. 3, is to be
Endoporeutic so that it is the outermost occurrence of
the Name that is the earliest. |
| |
|
|
| 569. |
Let us now
analyze the interpretation of a Ligature passing through
a Cut. Take, for example, the Graph of Fig 208. The
partial |
| |
 |
|
Figure 208 |
|
| |
Graph on the
Place of the Cut asserts that there exists an individual
denoted by the extremity of the line of identity on the
Cut, which is a millionaire. Call that individual C.
Then, since contiguous dots denote the same individual
objects, the extremity of the line of identity on the
Area of the cut is also C, and the Partial Graph on that
Area, asserts that, let the
Interpreter choose whatever individual he will, that
individual is either not C, or else is not unfortunate.
Thus, the Entire Graph asserts that there exists a
millionaire who is not unfortunate. Furthermore, the
Enclosure lying in the same Argent Province as the
"millionaire," it is asserted that this individual's
being a millionaire is connected with his not being
unfortunate. This example shows that the Graphist is
permitted to extend any Line of Identity on a recto Area
so as to carry an end of it to any Cut in that area. Let
us next interpret Fig. 209. It obviously asserts that
there exists a Turk who is at once the husband of an
Individual denoted by a point on the Cut, |
| |
 |
|
Figure 209 |
|
| |
which
individual we may name U, and is the husband of an
Individual, whom we may name V, denoted by another point
on the Cut. And the Graph on the Area of the cut,
declares that whatever Individual the Interpreter may
select either is not, and cannot be, U, or is not and
cannot be V. Thus, the Entire Graph asserts that there
is an existent Turk who is husband of two existent
persons; and the "husband," the "Turk" and the
enclosure, all being in the same Argent province,
although the Area of the Enclosure is on color, and thus
denies the possibility of the identity of U and V, all
four predications are true together, that is, are true
under the same circumstances, which circumstances should
be defined by a special convention when anything may
turn upon what they are For the sake of illustrating
this, I shall now scribe Fig. 210 all in one province.
This may be read "There is some married woman who will
commit |
| |
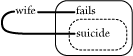 |
|
Figure 210 |
|
| |
suicide in
case her husband fails in business." This evidently goes
far beyond saying that if every married man fails in
business some married woman will commit suicide. Yet
note that since the Graph is on Metal it asserts a
conditional proposition de inesse and only means that
there is a married woman whose husband does not fail or
else she commits suicide. That, at
least, is all it will seem to mean if we fail to take
account of the fact, that being all in one Province, it
is said that her suicide is connected with his failure.
Neglecting that, the proposition only denies that every
married man fails, while no married woman commits
suicide.(*) The
logical Principle is that to say that there is some one
individual of which one or other of two predicates is
true is no more than to say that there either is some
individual of which one is true or else there is some
individual of which the other is true. Or to state the
matter as an illative permission of the System of
Existential Graphs,
Fourth Permission. If the smallest Cut which wholly
contains a Ligature connecting two Graphs in different
Provinces has its Area on the side of the Leaf opposite
to that of the Area of the smallest Cut that contains
those two Graphs, then such Ligature may be made or
broken at pleasure, as far as these two Graphs are
concerned.(**) |
| |
|
|
|
570. |
Another
somewhat curious problem concerning ligatures is to say
by what principle it is true, as it evidently is true,
that the passage of ligatures from without the outer of
two Cuts to within the inner of them will not prevent
the two from collapsing in case there is no other
Graph-Instance between them. A little study suffices to
show that this may depend upon the ligatures' being
replaceable by Selectives where they cross the Cuts, and
that a Selective is always, at its first occurrence, a
new predicate. For it is a principle of Logic that in
introducing a new predicate one has a right to assert
what one likes concerning it, without any restriction,
as long as one implies no assertion concerning anything
else. I will leave it to you, Reader, to find out how
this principle accounts for the collapse of the two
Cuts. Another solution of this problem, not depending on
the superfluous device of Selectives, is afforded by the
second enunciation of the Rule of Iteration and
Deiteration; since this permits the Graph of the Inner
Close to be at once iterated on the Phemic Sheet. One
may choose between these two methods of solution. |
| |
|
|
| 571. |
The System of
Existential Graphs which I have now
sufficiently described--or, at any rate, have described
as well as I know how, leaving the further perfection of
it to others--greatly facilitates the solution of
problems of Logic, as will be seen in the sequel, not by
any mysterious properties, but simply by substituting
for the symbols in which such problems present
themselves, concrete visual figures concerning which we
have merely to say whether or not they admit |
| |
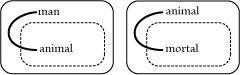 |
|
Figure 211 |
|
| |
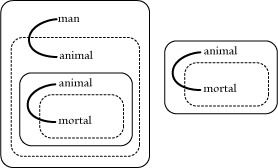 |
|
Figure 212 |
|
| |
certain
describable relations of their parts. Diagrammatic
reasoning is the only really fertile reasoning. If
logicians would only embrace this method, we should no
longer see attempts to base their science on the fragile
foundations of metaphysics or a psychology not based on.
logical theory; and there would soon be such an advance
in logic that every science would feel the benefit of
it. This System may of course be
applied to the analysis of reasonings. Thus to separate
the syllogistic illation, "Any man would be an animal,
and any animal would be mortal; therefore any man would
be mortal," the Premisses are first scribed as in Fig.
211. Then by the rule of Iteration, a first illative
transformation gives Fig. 212. Next, by the permission
to erase from a recto Area, a second step gives Fig.
213.
Then, by the permission to deform
a line of Identity on a recto Area, a third step gives
Fig. 214. Next, by the permission to insert in a verso
Area, a fourth step gives Fig. 215. Next, by
Deiteration, a fifth step gives Fig. 216. Next, by |
| |
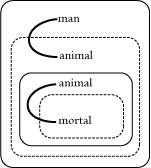 |
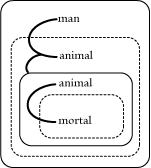 |
|
Figure 213 |
Figure 214 |
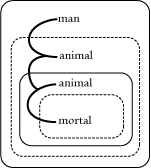 |
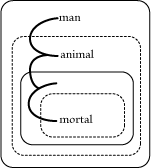 |
|
Figure 215 |
Figure 216 |
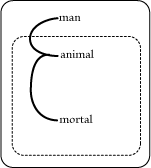 |
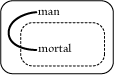 |
|
Figure 217 |
Figure 218 |
|
| |
the collapse
of two Cuts, a sixth step gives Fig. 217; and finally,
by omission from a recto Area, a seventh step gives the
conclusion Fig. 218. The analysis might have been
carried a little further, by means of the Rule of
Iteration and Deiteration, so as to
increase the number of distinct inferential steps to
nine, showing how complex a process the drawing of a
syllogistic conclusion really is. On the other hand, it
need scarcely be said that there are a number of deduced
liberties of transformation, by which even much more
complicated inferences than a syllogism can be performed
at a stroke. For that sort of problem, however, which
consists in drawing a conclusion or assuring oneself of
its correctness, this System is not particularly
adapted. Its true utility is in the assistance it
renders--the support to the mind, by furnishing concrete
diagrams upon which to experiment--in the solution of
the most difficult problems of logical theory. |
| |
|
|
| 572. |
I mentioned on
an early page of this paper that this System leads to a
different conception of the Proposition and Argument
from the traditional view that a Proposition is composed
of Names, and that an Argument is composed of
Propositions. It is a matter of insignificant detail
whether the term Argument be taken in the sense of the
Middle Term, in that of the Copulate of Premisses, in
that of the setting forth of Premisses and Conclusion,
or in that of the representation that the real facts
which the premisses assert (together it may be, with the
mode in which those facts have come to light) logically
signify the truth of the Conclusion. In any case, when
an Argument is brought before us, there is brought to
our notice (what appears so clearly in the Illative
Transformations of Graphs) a process whereby the
Premisses bring forth the Conclusion, not informing the
Interpreter of its Truth, but appealing to him to assent
thereto. This Process of Transformation, which is
evidently the kernel of the matter is no more built out
of Propositions than a motion is built out of positions.
The logical relation of the Conclusion to the Premisses
might be asserted; but that would not be an Argument,
which is essentially intended to be understood as
representing what it represents only in virtue of the
logical habit which would bring any logical Interpreter
to assent to it. We may express this by saying that the
Final (or quasi-intended) Interpretant of an Argument
represents it as representing its Object after the
manner of a Symbol. In an analogous way the relation of
Predicate to Subject which is stated in a Proposition
might be merely described in a Term. But
the essence of the Proposition is that it intends, as it
were, to be regarded as in an existential relation to
its Object, as an Index is, so that its assertion shall
be regarded as evidence of the fact. It appears to me
that an assertion and a command do not differ
essentially in the nature of their Final Interpretants
and in their Immediate, and so far as they are effective
in their Dynamical Interpretants; but that is of
secondary interest. The Name, or any Seme, is merely a
substitute for its Object in one or another capacity in
which respect it is all one with the Object. Its Final
Interpretant thus represents it as representing its
Object after the manner of an Icon by mere agreement in
idea. It thus appears that the difference between the
Term, the Proposition, and the Argument, is by no means
a difference of complexity, and does not so much consist
in structure as in the services they are severally
intended to perform. For
that reason, the ways in which Terms and Arguments can
be compounded cannot differ greatly from the ways in
which Propositions can be compounded. A mystery, or
paradox, has always overhung the question of the
Composition of Concepts. Namely, if two concepts, A and
B, are to be compounded, their composition would seem to
be necessarily a third ingredient, Concept C, and the
same difficulty will arise as to the Composition of A
and C. But the Method of Existential Graphs solves this
riddle instantly by showing that as far as propositions
go, and it must evidently be the same with Terms and
Arguments, there is but one general way in which their
Composition can possibly take place; namely each
component must be indeterminate in some respect or
another; and in their composition each determines the
other. On the recto this is obvious: "Some man is rich"
is composed of "Something is a man" and "something is
rich," and the two somethings merely explain each
other's vagueness in a measure. Two simultaneous
independent assertions are still connected in the same
manner; for each is in itself vague as to the Universe
or the "Province" in which its truth lies and the two
somewhat define each other in this respect. The
composition of a Conditional Proposition is to be
explained in the same way. The Antecedent is a Sign
which is Indefinite as to its Interpretant; the
Consequent is a Sign which is Indefinite as to its
Object. They supply each the other's lack. Of course,
the explanation of the structure of the Conditional
gives the explanation of negation; for the negative is
simply that from whose Truth it would be true to say
that anything you please would follow de inesse.
In my next paper, the utility of this
diagrammatization of thought in the discussion of the
truth of Pragmaticism shall be made to appear.(*) |
| |
|
|
|
|